1114 Lab 1: Introduction to 1114 Labs

Part 1 Measurements and uncertainty

1. The zero reading

What is the zero reading? Look at the picture of the kitchen scale on the right. You can click on the picture to enlarge it. With nothing in the tray, the reading should be 0 but it is not. We call this reading "the zero reading".

Before taking a measurement, with the tray empty, you should turn the white knob under the tray so that the reading is zero.

Digital devices may also need to be zeroed before taking a measurement, like this digital balance. This can be done by pressing the "zero" button on the balance.

There are two ways to solve the zero reading problem. Above is the first method, where you "zero the zero reading". You can also "correct your readings by the zero reading":

Look at the picture on the right, where we use a micrometer to measure the diameter of a glass marble. The zero reading (top picture) is −0.10 mm, and the reading (bottom picture) is 11.28 mm. So the correct reading is 11.28 mm − (−0.10 mm) = 11.38 mm. Because the micrometer has a negative zero reading (which is very common for micrometers), the corrected reading is in fact larger than the actual reading.

2. Calibrations

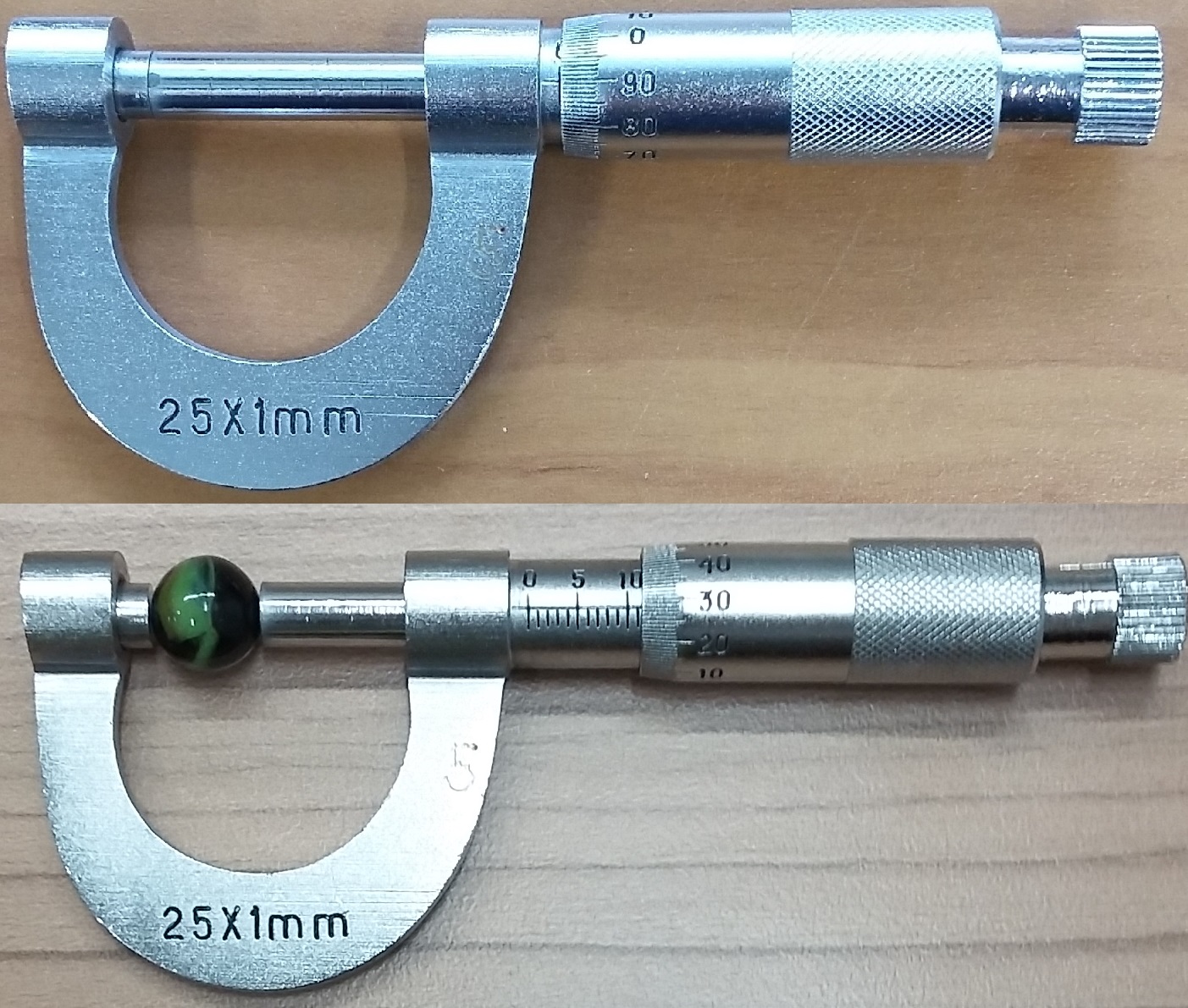
Another thing you must check before taking any measurements is the calibration. What does this mean? A measuring device, especially if it is old or home made, may not be showing the correct values. For example, the spring inside the kitchen scale may have lost its elasticity over time, then all the mass readings will be too big. To eleminate such errors, we need to calibrate the kitchen scale before use.

Calibrations need to be done with standard objects or values. For example, you can calibrate a thermometer with ice water and boiling water, calibrate an accelerometer with known gravity acceleration, calibrate a clock with the time on your cellphone, etc.

To calibrate our kitchen scale, we first check the zero reading, and then put a standard 1 kg mass in the tray. If the reading is 1000 g, then the scale is calibrated. Our picture shows a number that is a little bit more than 1000 g, which means this kitchen scale is a bit out of calibration, which we will have to live with.

To calibrate the digital balance, use the "CAL" button and a standard 200 g mass.

You may not have standard masses, but you can get something from the grocery store, like 1.00 kg of rice, to calibrate your own scale.

3. Recording a measurement

Most of our experiments begin with taking data. That is, measure some quantities and record the readings. You must always have these three key elements for a reading:

These digits are called significant digits, or significant figures, or sig figs.

Lets look at two examples:

You can read the hundreds digit and the tens digit exactly, because each small division is 10 g. You have to estimate the ones digit. If the pointer is between two division lines, you can estimate the number to be, say, 455 g. But here the pointer is almost on the division line, so you should record, in non-erasable ink, "The mass of the butter brick is 460 g." It has 3 sig figs where 0 is the estimated digit.

You should record, in non-erasable ink, "The mass of the butter brick is 459.3 g." It has 4 sig figs.

4. Recording with uncertainty

The last digit in the above readings is not exact: it has an uncertainty. In some labs we require you write the uncertainty explicitly, so that the reading looks like (460 ± 5)g. Here 5g is the uncertainty: not only it says that digit 0 is uncertain, but also the range of this measurement is from 455g to 465g.

For the digital balance, if we want to know the uncertainty, we need to look up the manual. By googling the model of the balance, one can find the manual at https://scaleman.com/vic-511-electronic-scale.html, and the "readability" of this scale is 0.1 g. So we can record the mass of the butter brick to be (459.3 ±0.1) g."

You should see that the uncertainty and the sig figs are closely related: the last sig fig is where the uncertainty lies:

The uncertainty also has the same units as the reading, and you should show that when recording the data. 460g ± 5g and (460 ± 5)g are both correct, but 460g ± 5 misses the units of the uncertainty.

How should we assign an uncertainty to a reading?

For reading an analog scale, assuming everything is stable and clear, we usually take half of the smallest division to be the uncertainty. As in the previous example, for reading the mass on the right, the uncertainty is 5g, half of its smallest division.

However, in many situations, there are other considerations. One common source of uncertainty is the parallax. When you move your head slightly in reading the height of the bowl on the right, the reading can change by a whole division.  Therefore, you should use 0.1 cm to be the uncertainty, and the reading should be (7.1 ± 0.1) cm.

Another common reason for large uncertainty is that the quantity being measured varies. For the butter brick on the right, the length at different place can change by as much as 3 divisions. So we can record the length to be (11.4 ± 0.2)cm.

There are some methods to decide the uncertainty, like repeating a measurement, but you will also learn from experiences. Uncertainty is not an exact number, so there is no unique answer. Whoever takes the measurement knows the uncertainty the best, so he/she is responsible to record the uncertainty.

We must remember that even if we do not write the uncertainty explicitly, the reading still has an uncertainty lying inside the last sig fig. If the last sig fig happens to be 0, you must not round it away: Although 2.5g and 2.50g are equal in size, they have different uncertainties and precision. 2.5g has 2 sig figs while 2.50g has 3 sig figs and is a more precise number.

(Leading zeros like zeros in 0.025g are not significant figures. They are related to the size of the number, but not related to the uncertainty.)

Part 2 Sig fig rules

Uncertainty is very important throughout the physical experiments, from taking data to reporting the final result. In higher level labs like PHYS1125, we will learn how to calculate the uncertainty of the result. But for 1114 and 1118, we do not calculate uncertainties. Instead, we use the significant figures to indicate the size of the uncertainty of the result. To find out the sig figs in a calculated result, let us learn two sig fig rules.

When using data to calculate the result, the precision of the result cannot be better than any data. In other words, the sig figs in the result is limited by the piece of data that is least precise. This is the general principle, but it takes different forms for different calculations. For multiplication and division, the more sig figs there are, the more precise a number is. Therefore:

However, when you add or subtract numbers, what matters to the precision is not how many sig figs there are, but rather where the last sig fig locates. The more it is to the left in a number, the bigger is the uncertainty. The rule is:

Let's walk through the examples in "Measurements: Basic" to see how to apply these two rules. We underscored the last sig fig to keep track of the sig figs in the process of calculations, but not for the final result. Please note that we keep more than significant digits for intermediate numbers to avoid getting different result due to rounding off.

Part 3 Writing a lab report

Please click here for instructions on how to write your first PHYS 1114 lab report.